No, the acceleration due to gravity is not directly proportional to the distance inside the Earth. In fact, the acceleration due to gravity decreases as you move towards the centre of the Earth.
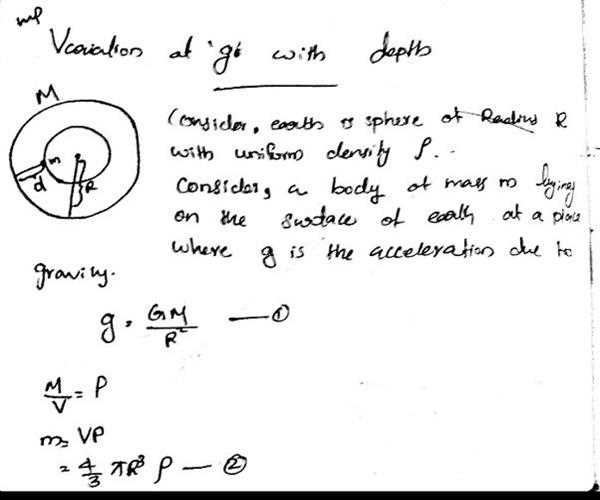
The acceleration due to gravity is calculated using the following formula:
Code:
g = G * M / r^2
where:
- g is the acceleration due to gravity
- G is the gravitational constant
- M is the mass of the Earth
- r is the distance from the centre of the Earth
As you can see from the formula, the acceleration due to gravity is inversely proportional to the square of the distance from the centre of the Earth. This means that as the distance from the centre of the Earth decreases, the acceleration due to gravity increases.
At the surface of the Earth, the acceleration due to gravity is about 9.8 m/s^2. However, at the centre of the Earth, the acceleration due to gravity is zero. This is because all of the mass of the Earth is evenly distributed around the centre, so there is no net gravitational force pulling you towards any one point.
The acceleration due to gravity also varies with the density of the Earth. The denser the Earth, the greater the acceleration due to gravity. This is because the gravitational force is proportional to the mass of the object, and the mass of the Earth is proportional to its density.
In conclusion, the acceleration due to gravity is not directly proportional to the distance inside the Earth. In fact, the acceleration due to gravity decreases as you move towards the centre of the Earth. The acceleration due to gravity also varies with the density of the Earth.